9 Mar 2014

Drag Cofficient - Definition, Equation & Examples.


Definition
In fluid dynamics, the drag coefficient (commonly denoted as: cd, cx or cw) is a dimensionless quantity that is used to quantify the drag or resistance of an object in a fluid environment such as air or water. It is used in the drag equation, where a lower drag coefficient indicates the object will have less aerodynamic or hydrodynamic drag. The drag coefficient is always associated with a particular surface area.
Computer drawing of an airliner. Drag coefficient equals drag divided by the density times the area times half the velocity squared.
image source- https://www.grc.nasa.gov/www/k-12/airplane/dragco.html
The drag coefficient of any object comprises the effects of the two basic contributors to fluid dynamic drag: skin friction and form drag. The drag coefficient of a lifting airfoil or hydrofoil also includes the effects of lift-induced drag. The drag coefficient of a complete structure such as an aircraft also includes the effects of interference drag.



14ilf1l.svgEquation
The drag coefficient 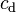c_\mathrm d\, is defined as:
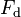c_\mathrm d = \dfrac{ F_\mathrm d}{ \dfrac{1}{2} \rho v^2 A}\,
where:
F_\mathrm d\, is the drag force, which is by definition the force component in the direction of the flow velocity,
\rho\, is the mass density of the fluid,
v\, is the speed of the object relative to the fluid and
A\, is the reference area.
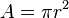The reference area depends on what type of drag coefficient is being measured. For automobiles and many other objects, the reference area is the projected frontal area of the vehicle. This may not necessarily be the cross sectional area of the vehicle, depending on where the cross section is taken. For example, for a sphere A = \pi r^2\, (note this is not the surface area = \!\ 4 \pi r^2).
For airfoils, the reference area is the planform area. Since this tends to be a rather large area compared to the projected frontal area, the resulting drag coefficients tend to be low: much lower than for a car with the same drag and frontal area, and at the same speed.
Airships and some bodies of revolution use the volumetric drag coefficient, in which the reference area is the square of the cube root of the airship volume. Submerged streamlined bodies use the wetted surface area.
Two objects having the same reference area moving at the same speed through a fluid will experience a drag force proportional to their respective drag coefficients. Coefficients for unstreamlined objects can be 1 or more, for streamlined objects much less.

Examples.
Aircraft use wing area as the reference area when computing c_\mathrm d\, , while automobiles (and many other objects) use frontal cross sectional area; thus, coefficients are not directly comparable between these classes of vehicles. In the aerospace industry the drag coefficient is sometimes expressed in drag counts where 1 drag count = 0.0001 of a C_d.


Aircraft
cdAircraft type
0.021F-4 Phantom (subsonic)
0.022Learjet 24
0.024Boeing 787
0.027Cessna 172/182
0.027Cessna 310
0.031Boeing 747
0.044F-4 Phantom II (supersonic)
0.048F-104 Starfighter
0.095X-15 (Not confirmed)